
Celestial Navigation is the art and science of using celestial bodies such as the Sun, Moon, planets and stars to determine an observer's position on Earth. This web page will explain the some of the math behind celestial navigation, and will provide the reader with a easy to use method determining position.
Contents:
1. The Celestial Sphere
2. The Astronomical Triangle
3. Lines of Position
4. References and Postscript Code

The Celestial Sphere is an essential concept of celestial navigation. Figure 1 depicts a celestial sphere centered on an observer on Earth. For our purposes the celestial sphere can be constructed of arbitray radius, here shown to be AK. Some definitions that will come in handy later on are given below.
Plumb Line: If we take the Earth (shown in brown) to be a sphere, then the radius AC represents the plumb line direction for the observer.
Zenith: The point directly above the observer on the celestial sphere is called the Zenith. The point opposite the zenith, or directly below the observer on the celestial sphere is called the nadir (not shown here).
Observer's Horizon: The plane tangent to the earth's surface at A (shown in pink) and perpendicular to the plumb line is the plane of the true or mathematical horizon of the observer.
Compass Points: The line NS and the line perpendicular to NS along E determine the main directions or the points of the compass for the observer.
Celestial Projection: The straight line AC' represents the direction to a celestial body C'. cf is the projection of C' on the celestial sphere.
Celestial Equator: The plane passing through the celestial sphere that is parallel to Earth's equater is the Celestial Equator (K is on the celestial equator in Figure 1).
Celestial axis and poles: The axis through the center of the celestial sphere perpendicular to the celestial equator is the celestial axis. The poles are at either end of this axis, corresponding to the Earth's poles (PN is the celestial north pole, Pn is the actual north pole).
Elevated Pole: The celestial pole that corresponds to the earth pole that is in the same hemisphere (N or S) as the observer. In Figure 1, PN is the elevated pole.
Vertical Circles: Circles passing through the zenith and nadir points are called Verticle Circles.
Meridians: Circles passing through both celestial poles are called Celestial Meridians, they correspond to meridians on the Earth (lines of longitude) which pass through both the north and south pole. The observer's meridian on the celestial sphere is the circle passing through KZPN.
Hour Angles: Hour angles are the spherical angle, or the arc of the celestial equator between two meridians. The local hour angle of the body C' above is the arc between the observer's meridian and the meridian passing through cf. Local hour angles are denoted tloc
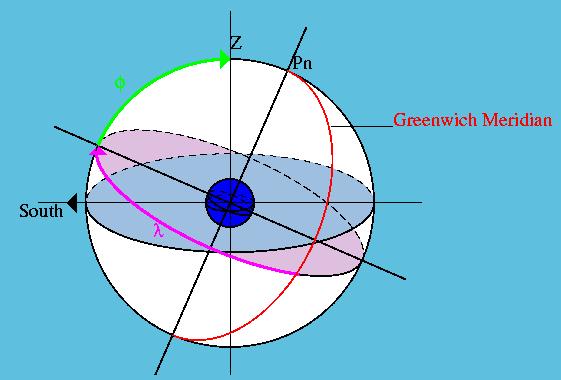
If the celestial sphere is drawn to be centered at the center of Earth then the latitude (f)and longitude (l) of the observer can be found by using the celestial sphere. Latitude is the arc or angle subtended from the closest point on the equator to the observer's zenith. The latitude is followed with a letter N or S depending which hemisphere the observer's meridian is located in.
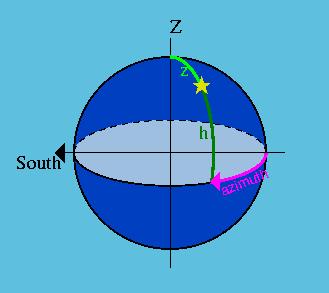
Two useful coordinates of a particular location are the Azimuth (A) and the Altitude (h). If we have a celestial body (denoted by the yellow star in Figure 3) on the celestial sphere, then it's azimuth is the arc of the celestial horizon which lies between the observer's meridian and the vertical circle of the body. A can be given in a few ways. For our purposes A should be measured from the north or south points towards east or west, whichever gives
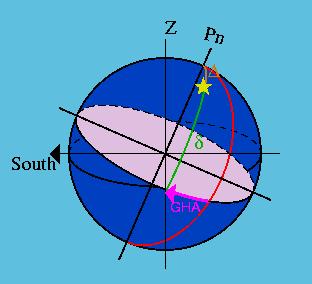
Two other important coordinates are declination (d) and the Greenwich Hour Angle (GHA). The declination of the celestial body (the body is denoted by star on Figure 4) is the arc of the body's meridian from the celestial equator to the place of the body on the sphere. In this respects the declination and latitude of the body are similar. However:
The Greenwich Hour Angle is the arc on the celestial equator between the Greenwich Meridian and the celestial meridian of the body. It is called the hour angle because due to earth's rotation, 15 degrees of longitude corresponds to 1 hour. ie: if the celestial sphere remained stationary and centered on earth while the earth rotated, the GHA of the celestial body would increase by 15 degrees every hour.