Interference of Light Waves - Young's Double-slit Experiment

1. Wave Nature of Light
2. Concepts
3. Parameters and Equations
4. Effects of Varying Certain Variables

Wave Nature of Light

Early physicists such as Issac Newton and Christiann Huygens thought tt was a steady stream of particles and had a wave motion. Indeed, such theories were correct but were unproven because light does indeed consists of moving quantas of photons which are packets of particles of pure energy. It was not until 1802, that Thomas Young proved that light does have a wave motion. In his experiment, he hypothesized that parallel beams of light should produce a brighter area where they overlapped if light were a steady stream. Instead, they produced bright bands separated by dark bands, the result of two sets of waves coinciding or canceling each other out. Thus, light is a form of wave.

Concepts

• Interference is the phenomenon that arises when two or more waves meet at the same place and their amplitudes add in a way that they are either constructive or destructive depending on their relative phases.
• Intereference occurs any time two waves interact, but in order to get a reliable pattern of intereference, coherent or zmonochromatic light sources are needed. This is because coherent light means that the waves emitted are in-phase, whereas monochromatic light emit a single color which means the waves are in-phase for sure. (This is why light bulbs are not used in light waves experiments because they are incoherent.)
• In his experiment, Young allowed sunlight to pass through a small hole, that in turn pass through a pair of closely spaced slits, which then illuminated a screen. Waves diffract at each slit and then interfere in the region between the slits and the screen thus causing a pattern of alternating dark and bright regions on the screen. These regions are called fringes.
• Dark fringes are the result of destructive interference when the waves are out of phase, whereas bright fringes are formed by constructive interence when the waves are in-phase.

Parameters and Equations

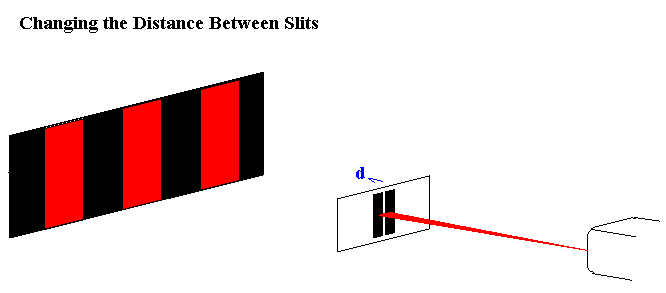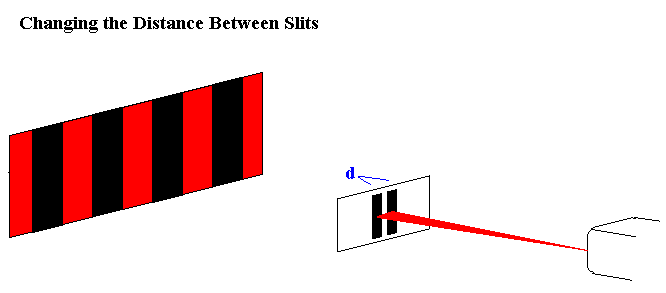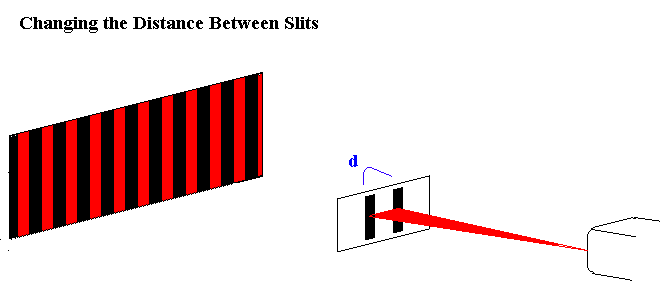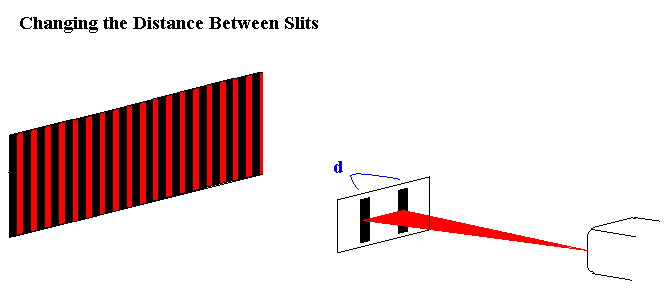
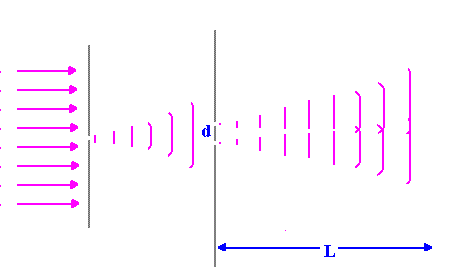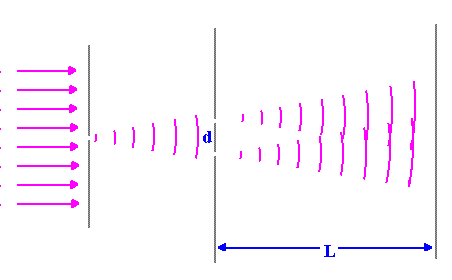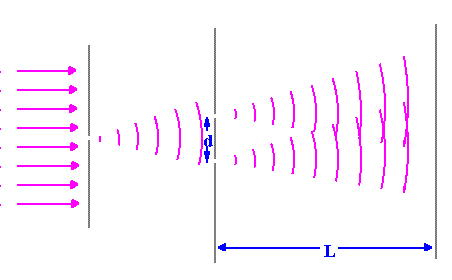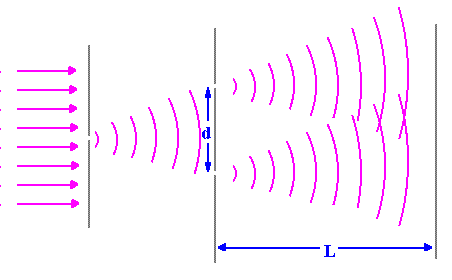
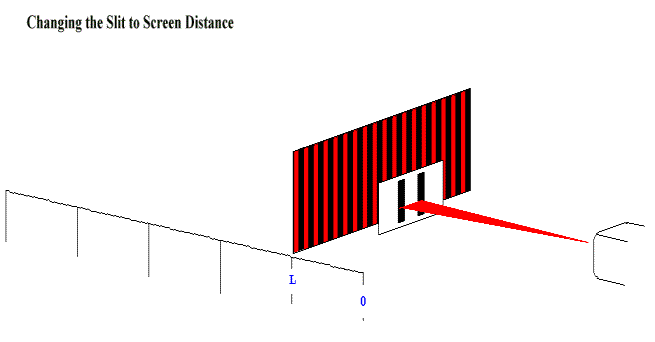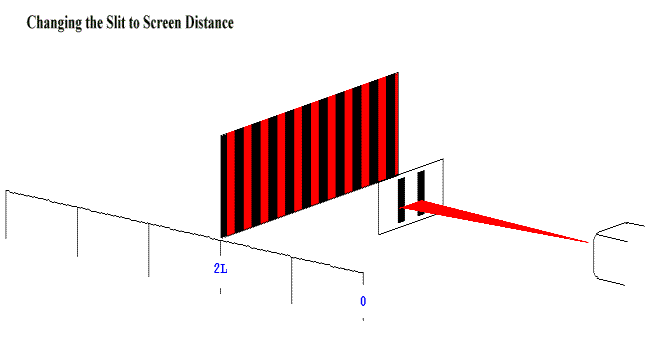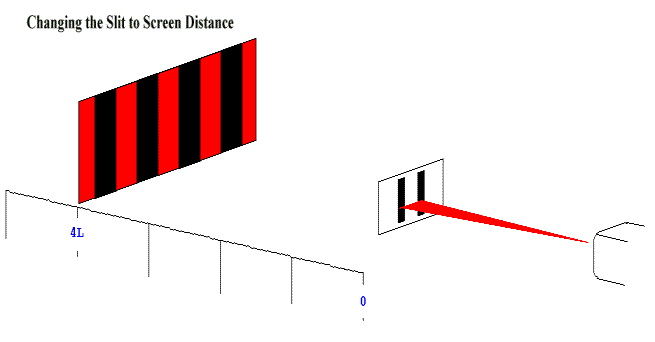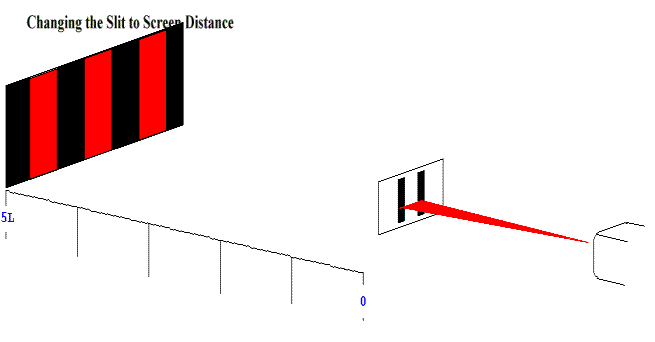
Parameters d is the distance between the 2 slits y is the vertical distance from the center of the screen to the position of the fringe θ is the angle made by a line to P from the point midway between the slits L is the distance between the slits and the screen r1 is the first path r2 is the second path δ is the path difference m is the order number of the fringe S1 is the first slit S2 is the second slit

Effects of Varying Certain Variables

Varying d Increasing the length of d decreases the spacing between different fringes. This is consistent with the fact that spacings between different fringes inversely depend on d. This second diagram shows what happens to waves as they pass through the slits as d varies. More interference occurs when d is wider.
Varying L The spacings between different fringes decreases as the distance between the slits increases because it is dependent on L.
Varying the Wavelength Increasing the wavelength of the light increases the spacing between different fringes since the spacing between different fringes is wavelength dependent. Red light has the largest wavelength of the color spectrum with a range of 625 - 740 nm, while violet has the smallest wavelength with a range of 380 - 435 nm.