Hyperbolic 3-Manifolds

Math 603D

Fall 2021

This is a course about 3-manifolds and hyperbolic geometry. We will start by understanding some of the key theorems underlying the current study of geometric topology in dimension 3; see [Po2019]. This will follow Thurston's original notes [Th1980] to some degree, but we will suplement with the relevant chapters of Ratcliffe's book [Ra2006]. From there, the course will go in one of (at least) two possible directions: towards the study of character varieties (with some of [CAM2020] as a goal) or in the direction of essential surfaces and mapping class groups (with some of [HS2009] as a goal). Further references will be added as the course proceeds.

As a starting point, this course will assume familiarity with knot theory and three-manifolds. A great reference for this is Dale Rolfsen's classic book Knots and Links [Ro1976].

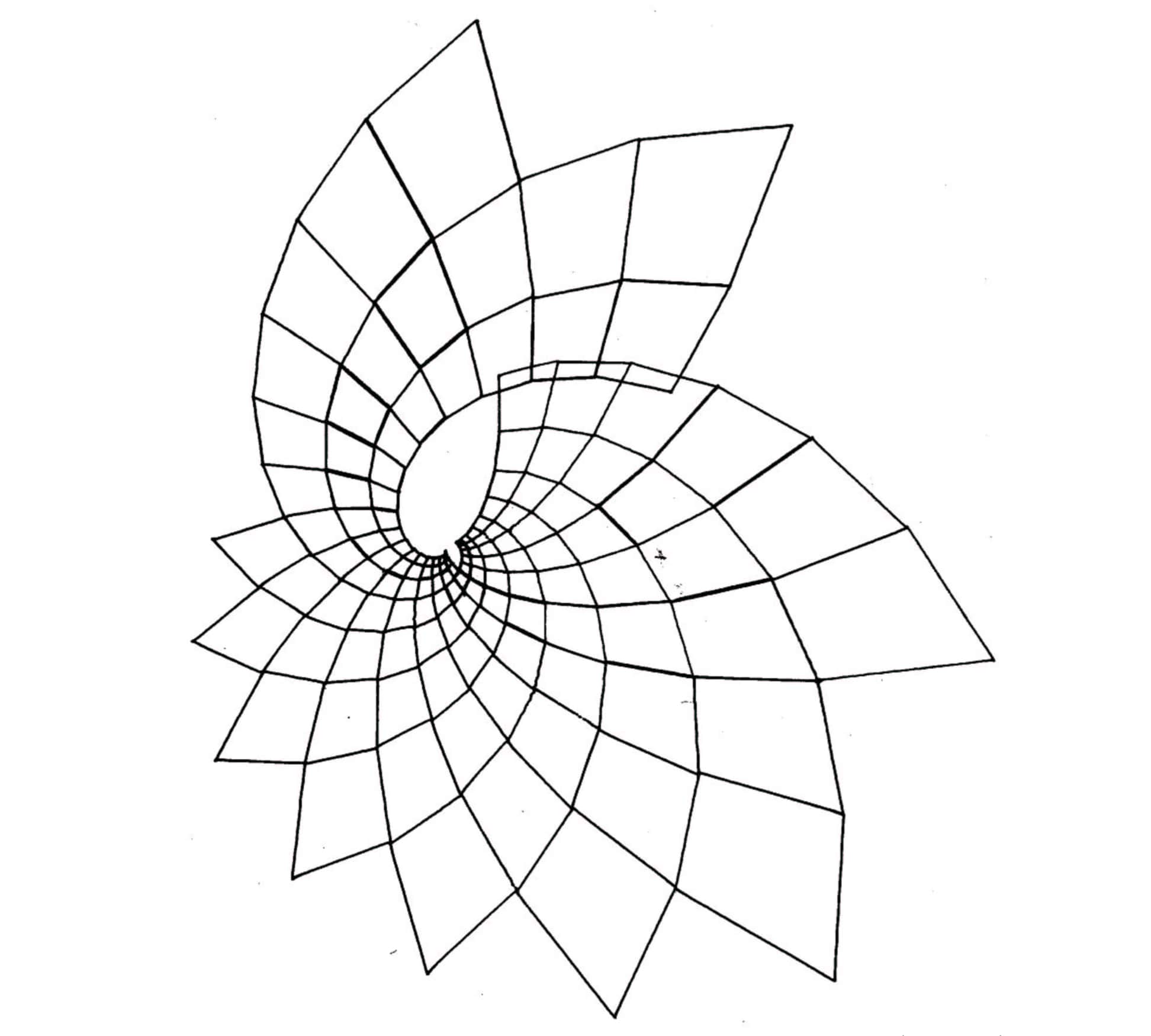
The image on the right is borrowed from Thurston's famous notes based on courses he gave at Princeton in the early 1980s [Th1980].

• Instructor
  Liam Watson
  liam(at)math(dot)ubc(dot)ca

• Where, when...
  The class meets Mondays and Wednesdays 12:00 to 2:00. The first meeting is on September 8, 2020.

• Evaluation
  This is a participating seminar. Your final grade will be entirely based on the presentations you give as part of the course.

• Resources
  As a starting point, we will make use of Ratcliffe's book Foundations of hyperbolic manifolds [Ra2006], for which you can get a free digital copy though the UBC library (linked here).