- Nanoparticles through airway mucus
- Hydrogel-liquid interfaces
- Epithelial tissue morphogenesis
- Interfacial dynamics in complex fluids
- Cell mechanics, chemotaxis and collective migration
- Tear film breakup in healthy and infected eyes
(in collaboration with Pengtao Yue of Virginia Tech and Yuan-nan Young of NJIT)
Hydrogels are crosslinked polymer networks swollen with an aqueous solvent. As soft solids mechanically and chemically compatible with living cells, hydrogels are often used in microfluidic and organ-on-chip devices as carrier matrix for cell cultures.
In most of these applications, hydrogels are deployed using flowing liquids, thus giving rise to a fluid-gel two-phase flow situations. How does a hydrogel interact mechanically with a flowing fluid? How to use fluid dynamics to control the gel-fluid interface for optimal configurations? Such questions have inspired our research on the interfacial dynamics of hydrogels.
Our first task is to develop a theoretical formulation, with proper boundary conditions (BCs) on the interface between a hydrogel and a clear fluid. Adopting a poroelasticity model for the gel, we must supply additional BCs to specify the velocity jumps at the fluid-gel interface. This has been achieved by a thermodynamic argument of positive entropy production on the interface. Thus, we have proposed 3 different sets of BCs and compared their performance in a series of test problems. The results point to one particular set as the best.
Using the above theoretical formulation, we have developed a finite-element algorithm for computing two-phase flows involving a hydrogel surrounded by a clear fluid. Currently, the numerical tool is being applied to several problems of fundamental and applied interest, including the flow around a gel particle, interfacial dynamics in microfluidics, and intracellular dynamics.
As an example, the following plots show a gel particle being deformed by a biaxial extensional flow, with the formation of cusps at the two poles under strong stretch (more details here):
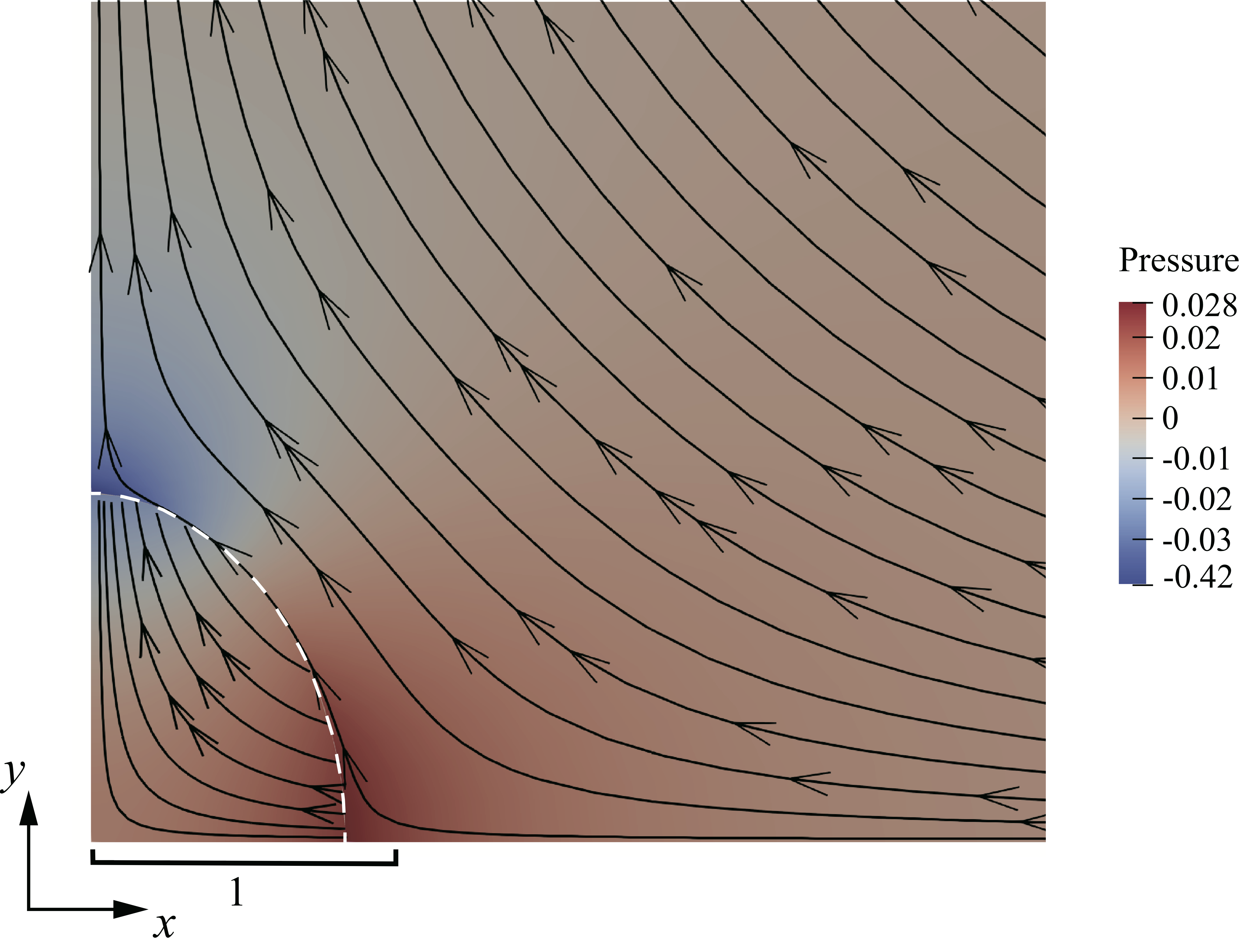
(Flow and pressure fields around a gel particle. For symmetry, only 1/4 of the particle is shown.)
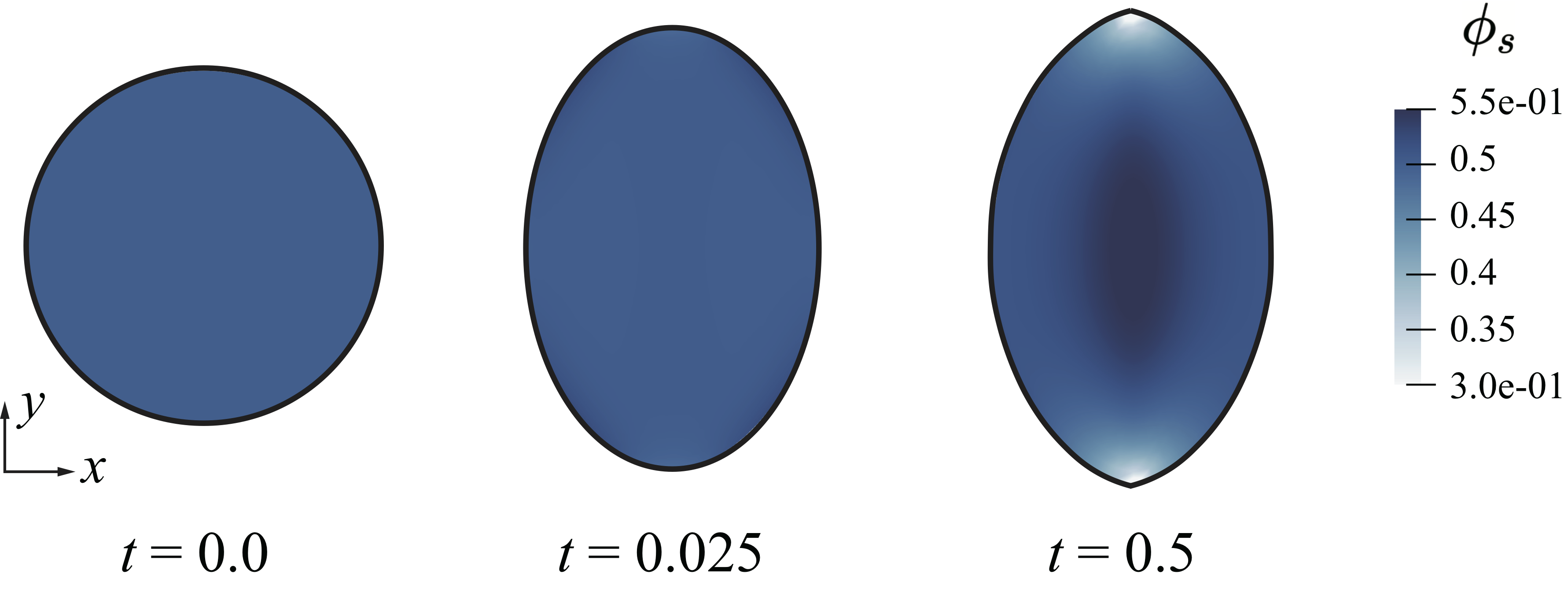
(Subject to stronger flow, the gel particle develops sharp points at its north and south poles, where the solid network is stretched to a very low volume fraction.)