arbelos   What is an arbelos? Properties of an arbelos         Distances and areas         Archimedes' circles         Apollonius circle         Bankoff circle         Pappus chain References   Stephen Tan 42373027 Dec 16, 2005 Math 308 by Dr. Bill Casselman Final Project Page 1 Label the diameters of the left and right semicircles r and 1 - r, so that the enclosing semicircle has diameter 1. 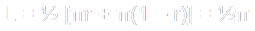The arc length along the bottom of the arbelos is: The arc length along the two smaller semicircles is the same as the arc length of the enclosing semicircle. 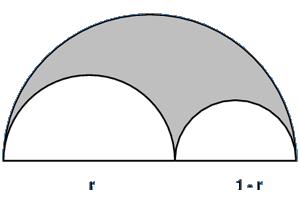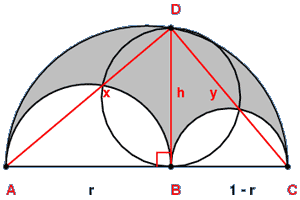Archimedes' Book of Lemmas, Proposition IV Let D be any point on a semicircle of diameter AC, and let BD be perpendicular to AC. If semicircles be described within the first semicircle and having AB and BC as diameters respectively, the figure included between the circumferences of the three semicircles is "what Archimedes called arbelos". Then the area of the arbelos is the same as the area of the circle with diameter BD.   Page 2 Let B be the point at the notch of the arbelos. By drawing a perpendicular line BD to the circumference of the enclosing circle, the area of the circle with the diameter BD is equal to the area of the arbelos.
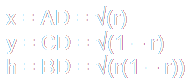